Tożsamości trygonometryczne – podstawowe zależności pomiędzy funkcjami trygonometrycznymi.
Tożsamości pitagorejskie
 Osobny artykuł: jedynka trygonometryczna.
Osobny artykuł: jedynka trygonometryczna.
Wzór

jest prawdziwy dla dowolnej liczby rzeczywistej (a nawet zespolonej, przy przyjęciu ogólniejszych definicji). Tożsamość ta uznawana jest za podstawową tożsamość trygonometryczną. Zwana często jedynką trygonometryczną bądź trygonometrycznym twierdzeniem Pitagorasa.
Istnieją również dwie inne wariacje tego wzoru:


Okresowość funkcji
Funkcje trygonometryczne są okresowe 

Definicje tangensa i cotangensa



Przedstawienia przy pomocy funkcji cosinus



Przedstawienia przy pomocy funkcji sinus



Parzystość i nieparzystość funkcji trygonometrycznych

Zależności pomiędzy funkcjami a kofunkcjami
Równości
![{\displaystyle {\begin{aligned}&\sin x=\cos \left({\frac {\pi }{2}}-x\right)&&\cos x=\sin \left({\frac {\pi }{2}}-x\right)\\[.2em]&\operatorname {tg} x=\operatorname {ctg} \left({\frac {\pi }{2}}-x\right)&&\operatorname {ctg} x=\operatorname {tg} \left({\frac {\pi }{2}}-x\right)\\[.2em]&\sec x=\operatorname {cosec} \left({\frac {\pi }{2}}-x\right)&&\operatorname {cosec} x=\sec \left({\frac {\pi }{2}}-x\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bfd95bdb88e651940e908933f83dcad199334479)
nazywa się związkami pomiędzy funkcjami a ich kofunkcjami. Kofunkcją sinusa jest cosinus, cosinusa sinus, tangensa cotangens itd.
Odwrotności
Funkcje trygonometryczne można układać w pary według kofunkcji lub według odwrotności. Odwrotnością sinusa jest cosecans, cosinusa secans, tangensa cotangens (i oczywiście na odwrót):
![{\displaystyle {\begin{aligned}&\sin x={\frac {1}{\operatorname {cosec} x}}&&\operatorname {cosec} x={\frac {1}{\sin x}}\\[.5em]&\cos x={\frac {1}{\sec x}}&&\sec x={\frac {1}{\cos x}}\\[.5em]&\operatorname {tg} x={\frac {1}{\operatorname {ctg} x}}&&\operatorname {ctg} x={\frac {1}{\operatorname {tg} x}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c997a622134bd906219470948bb3121aa2633ce5)
Funkcje sumy i różnicy kątów




Funkcje wielokrotności kątów
Wzory na dwukrotność kąta otrzymuje się przez podstawienie  we wzorach na funkcje sumy kątów.
we wzorach na funkcje sumy kątów.












Ogólnie:
![{\displaystyle {\begin{aligned}\sin nx&=\sum _{i=0}^{\infty }(-1)^{i}\cdot {n \choose 2i+1}\cos ^{n-2i-1}x\sin ^{2i+1}x\\[2pt]&=\sum _{i=0}^{\lfloor {\frac {n}{2}}\rfloor }(-1)^{i}\cdot {n \choose 2i+1}\cos ^{n-2i-1}x\sin ^{2i+1}x\\&=n\cos ^{n-1}x\sin x-{n \choose 3}\cos ^{n-3}x\sin ^{3}x+{n \choose 5}\cos ^{n-5}x\sin ^{5}x-{n \choose 7}\cos ^{n-7}x\sin ^{7}x+\dots \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/714e1218d101c47c2664961856dd7d3326ba7738)
![{\displaystyle {\begin{aligned}\cos nx&=\sum _{i=0}^{\infty }(-1)^{i}\cdot {n \choose 2i}\cos ^{n-2i}x\sin ^{2i}x\\[2pt]&=\sum _{i=0}^{\lfloor {\frac {n}{2}}\rfloor }(-1)^{i}\cdot {n \choose 2i}\cos ^{n-2i}x\sin ^{2i}x\\&=\cos ^{n}x-{n \choose 2}\cos ^{n-2}x\sin ^{2}x+{n \choose 4}\cos ^{n-4}x\sin ^{4}x-{n \choose 6}\cos ^{n-6}x\sin ^{6}x+\dots \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3eb40d217ed38a880497203d0468cb9a544202f5)

Funkcje kąta połówkowego






Suma i różnica funkcji











Iloczyn w postaci sumy







Potęgi w postaci sumy








Funkcje trygonometryczne wyrażone przy pomocy tangensa połowy kąta



Powyższe tożsamości znalazły zastosowanie w tzw. podstawieniu uniwersalnym, stosowanym przy obliczaniu całek typu  gdzie
gdzie  jest funkcją wymierną zmiennych
jest funkcją wymierną zmiennych  Stosuje się podstawienie:
Stosuje się podstawienie:



Wzory Eulera





Wzory te pozwalają łatwo przekształcać wyrażenia trygonometryczne, poprzez przejście na postać zespoloną (cztery ostatnie wzory), uproszczenie i powrót na postać trygonometryczną (pierwszy wzór).
Inne zależności między funkcjami trygonometrycznymi

Wzór de Moivre’a

lub ogólniej:
![{\displaystyle [r(\cos x+i\sin x)]^{n}=r^{n}(\cos nx+i\sin nx)\qquad n\in \mathbb {N} }](https://wikimedia.org/api/rest_v1/media/math/render/svg/76357c43423817aec6638c42c3dfecbcfbbcfde5)
Zobacz też
- trygonometryczne wzory redukcyjne
 Osobny artykuł: jedynka trygonometryczna.
Osobny artykuł: jedynka trygonometryczna.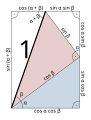 Sinus i cosinus sumy kątów
Sinus i cosinus sumy kątów Sinus i cosinus różnicy kątów
Sinus i cosinus różnicy kątów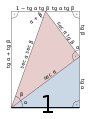 Tangens sumy kątów
Tangens sumy kątów Tangens różnicy kątów
Tangens różnicy kątów Cotangens sumy kątów
Cotangens sumy kątów Cotangens różnicy kątów
Cotangens różnicy kątów Osobny artykuł: Wzór Eulera.
Osobny artykuł: Wzór Eulera.














![{\displaystyle {\begin{aligned}&\sin x=\cos \left({\frac {\pi }{2}}-x\right)&&\cos x=\sin \left({\frac {\pi }{2}}-x\right)\\[.2em]&\operatorname {tg} x=\operatorname {ctg} \left({\frac {\pi }{2}}-x\right)&&\operatorname {ctg} x=\operatorname {tg} \left({\frac {\pi }{2}}-x\right)\\[.2em]&\sec x=\operatorname {cosec} \left({\frac {\pi }{2}}-x\right)&&\operatorname {cosec} x=\sec \left({\frac {\pi }{2}}-x\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bfd95bdb88e651940e908933f83dcad199334479)
![{\displaystyle {\begin{aligned}&\sin x={\frac {1}{\operatorname {cosec} x}}&&\operatorname {cosec} x={\frac {1}{\sin x}}\\[.5em]&\cos x={\frac {1}{\sec x}}&&\sec x={\frac {1}{\cos x}}\\[.5em]&\operatorname {tg} x={\frac {1}{\operatorname {ctg} x}}&&\operatorname {ctg} x={\frac {1}{\operatorname {tg} x}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c997a622134bd906219470948bb3121aa2633ce5)























![{\displaystyle {\begin{aligned}\sin nx&=\sum _{i=0}^{\infty }(-1)^{i}\cdot {n \choose 2i+1}\cos ^{n-2i-1}x\sin ^{2i+1}x\\[2pt]&=\sum _{i=0}^{\lfloor {\frac {n}{2}}\rfloor }(-1)^{i}\cdot {n \choose 2i+1}\cos ^{n-2i-1}x\sin ^{2i+1}x\\&=n\cos ^{n-1}x\sin x-{n \choose 3}\cos ^{n-3}x\sin ^{3}x+{n \choose 5}\cos ^{n-5}x\sin ^{5}x-{n \choose 7}\cos ^{n-7}x\sin ^{7}x+\dots \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/714e1218d101c47c2664961856dd7d3326ba7738)
![{\displaystyle {\begin{aligned}\cos nx&=\sum _{i=0}^{\infty }(-1)^{i}\cdot {n \choose 2i}\cos ^{n-2i}x\sin ^{2i}x\\[2pt]&=\sum _{i=0}^{\lfloor {\frac {n}{2}}\rfloor }(-1)^{i}\cdot {n \choose 2i}\cos ^{n-2i}x\sin ^{2i}x\\&=\cos ^{n}x-{n \choose 2}\cos ^{n-2}x\sin ^{2}x+{n \choose 4}\cos ^{n-4}x\sin ^{4}x-{n \choose 6}\cos ^{n-6}x\sin ^{6}x+\dots \end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3eb40d217ed38a880497203d0468cb9a544202f5)

















































![{\displaystyle [r(\cos x+i\sin x)]^{n}=r^{n}(\cos nx+i\sin nx)\qquad n\in \mathbb {N} }](https://wikimedia.org/api/rest_v1/media/math/render/svg/76357c43423817aec6638c42c3dfecbcfbbcfde5)







